Page 1571 - MISUMI Thailand Economy Series

[Technical Data] Quantifiers, Unit Symbols, Chemical Symbols and Symbols of Elements Excerpts from JIS Z 8202 [Technical Data]
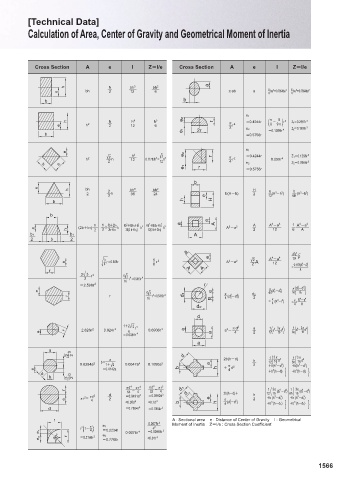
Calculation of Cubic Volume and Weight / Physical Properties of Materials Calculation of Area, Center of Gravity and Geometrical Moment of Inertia

■Name of Elements and Atomic Symbols
Atomic
Number Name Symbol Cross Section A e I Z＝I/e Cross Section A e I Z＝I/e
53 Iodine I
54 Xenon Xe
55 Cesium Cs
56 Barium Ba
57 Lanthanum La h h bh 3 bh 2 a Q Q
2
58 Cerium Ce bh 2 12 6 Qab a ba ＝0.7854ba 3 ba ＝0.7854ba 2
3
59 Praseodymium Pr e 4 4
60 Neodymium Nd b
61 Promethium Pm b
62 Samarium Sm
63 Europium Eu
64 Gadolinium Gd
65 Terbium Tb 2 e 1 Q
8
66 Dysprosium Dy h h h 4 h 3 e r ＝0.4244r ( 8 － 9Q) r 4 Z 1＝0.2587r 3
67 Holmium Ho e h 2 2 12 6 Q 2 r
2
68 Erbium Er 1 2r e 2 ＝0.1098r 4 Z 2＝0.1908r 3
69 Thulium Tm h e
70 Ytterbium Yb ＝0.5756r
71 Lutetium Lu
72 Hafnium Hf
73 Tantalum Ta
74 Tungsten W e e 1
75 Rhenium Re 2 h 4 2 2 e r ＝0.4244r Z 1＝0.1296r 3
3
76 Osmium Os h 2 2 h 12 0.11793h ＝ h 3 Q 2 r 0.055r 4
4
12
77 Iridium Ir h h e 2 Z 2＝0.0956r 3
78 Platinum Pt 1 e r ＝0.5756r
79 Gold Au
80 Mercury Hg
81 Thallium Tl
82 Lead Pb b
83 Bismuth Bi
84 Polonium Po e h bh 2 bh 3 bh 2 H b b
3
3
85 Astatine At 2 3 h 36 24 b(H－h) 2 12 (H －h ) 3 6H (H －h ) 3
86 Radon Rn e
87 Francium Fr b h H
88 Radium Ra
89 Actinium Ac
90 Thorium Th
91 Protactinium Pa b
92 Uranium U e
93 Neptunium Np h 1 3b＋2b1 6b ＋6bb 1＋b 1 2 3 6b ＋6bb 1＋b 1 2 2 a A 2 2 A A －a 4 1 A －a 4
4
4
2
2
94 Plutonium Pu e h (2b＋b1) 2 3 M 2b＋b1 h 36(2 b＋b 1) h 12(3 b＋2b1) h A －a 2 12 6 A
95 Americium Am
96 Curium Cm b1 1 b A
97 Berkelium Bk 2 b 2
98 Californium Cf
99 Einsteinium Es
100 Fermium Fm a 4 4
101 Mendelevium Md A -a 2
4
102 Nobelium No 3 5 3 e 2 2 2 A －a 4 12A
103 Lawrencium Lr e 4 r ＝0.866r 8 r A －a 2 A 12 0.1179(A －a ) 4
4
A A ＝ A
Reference This table is based on Appendix A(Names and Symbols of Elements)of r 3 3
ISO 31/8-1980(Amounts and Units of Physical Chemistry and Molecular 2 r 2 5 3 r ＝0.5413r 4
4
Physics) and Appendix C(Names and Symbols of Radionuclides) of ISO 2 16
31/9-1980(Amounts and Units of Atomic Physics and Nuclear Physics). ＝2.598r r Q 32( d 2 ) 4
4
2
4
e 5 3 e Q 64 (d －d ) 1 4 Q d －d 1
2
r r ＝0.5413r 3 d1 2 2 d 2
3
r
2
16 R 4 (d －d ) 1 2 Q 4 4
Q R －r
4
＝ (R －r ) 4 ＝ ×
4
d2 4 R
d
r
1＋2 2 4 Qd 2 a 1 1 4 3Q 4
4 3Q 4
2
d
d
r 2.828r 2 0.924r 2 6 0.6906r 3 e a － 12( a － ) 6a( a － 16 )
e a 4 2 16
＝0.6381r 4
a
a
b
2 2 d 1 3Q 1 3Q
a 2b(h－d) h 12{ 16 d 4 6h{ 16 d 4
0.8284a 2 b＝ 1＋ 2 0.0547a 4 0.1095a 3 e Q 2 2 ＋b(h －d ) 3 ＋b(h －d ) 3
3
3
3
3
e ＝0.4142a b h ＋ 4 d ＋b (h－d) } ＋b (h－d) }
b
b 2 b 2
Qd 4 Qr 4 Qd 3 Qr 3 d1 1 3 Q 1 3 Q
4
4
＝
■How to Calculate the Weight r 2 d 64 ＝ 4 4 ＝0.0982d 4 3 d 2b(h－d)＋ h 12 { 16 (d 1 －d ) 4 6h { 16 (d 1 －d ) 4
32
3
3

＝
Weight W[g]= Volume[cm 3 ]× Specific Gravity e Qr 2 Qd 4 2 ＝0.0491d e Q 2 2 2 ＋b (h －d 1) 3 ＋b (h －d 1) 3
≈0.05d 4 ≈0.1d 3 b h 4 (d －d ) ＋b (h－d 1) } ＋b (h－d 1) }
3
3
1
[Ex.]Material:Mild Steel d ＝0.7854r 4 ＝0.7854r 3
D=Ø16 L=50mm the weight is:
r 4 A : Sectional area e : Distance of Center of Gravity I : Geometrical
FD＝16 L＝50mm the weight is: r ( 1－ ) e 1 0.0075r Moment of Inertia Z＝I/e : Cross Section Coefficient
Q
2
e
2
e1 4 ＝0.2234r 0.0075r 4 ＝0.00966r 3
Q 2 90° r ＝0.2146r 2 e 2 3
The specific gravity of W ＝ 4 D ×L×W 2 e ＝0.7766r ≈0.01r
Q 2
＝ 4 ×1.6 ×5×7.85
≈79[g]
1565 1566
KJTIV JOEC

KJTIV JOEC